



Next: 4.3.2 The 2-leg ladder
Up: 4.3 SR measurements
Previous: Longitudinal-field measurements
We compare in Table 3 the
ordering temperatures of the Sr(Ca)-Cu-O series of compounds, which is
a one-dimensional chain system for Sr2CuO3 [55], and
a two-dimensional layer system for Ca0.86Sr0.14CuO2 (n
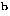 structure of the spin-ladder series)
[56]. The ordering temperature of the 3-leg ladder
system is between those of the chain system (Sr2CuO3) and
the layer system (Ca0.86Sr0.14CuO2). Suppression of
structure of the spin-ladder series)
[56]. The ordering temperature of the 3-leg ladder
system is between those of the chain system (Sr2CuO3) and
the layer system (Ca0.86Sr0.14CuO2). Suppression of
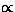 in the chain system has been explained as an effect of the
low-dimensionality [55]; the intermediate ordering
temperature for the 3-leg system is consistent with this idea, because the
3-leg ladder structure is the smallest extension of the
one-dimensional chain toward two-dimensionality.
in the chain system has been explained as an effect of the
low-dimensionality [55]; the intermediate ordering
temperature for the 3-leg system is consistent with this idea, because the
3-leg ladder structure is the smallest extension of the
one-dimensional chain toward two-dimensionality.
Table 3:
Structure and magnetic behavior of Sr(Ca)-Cu-O compounds
| compound |
structure |
magnetic order |
| Sr2CuO3 |
CuO chain (1D) |
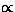 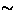 5 K 5 K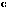 |
| Sr4Cu6O10 |
3-leg ladder |
T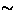 52 52 5 K 5 K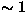 |
| Ca0.86Sr0.14CuO2 |
CuO2 plain (2D) |
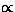 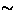 540 K 540 K |
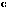 Obtained by
Obtained by  SR [55].
SR [55].
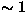 Obtained by
Obtained by  SR: this work [1].
SR: this work [1].
 Obtained by neutron scattering [57] and
Obtained by neutron scattering [57] and  SR [55].
SR [55].
Another feature of the magnetic order in the 3-leg ladder system is
the absence of critical slowing down of the Cu moments in the  SR time window (
SR time window (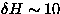 s). In Fig.31,
we show
s). In Fig.31,
we show  SR spectra at long times. No enhancement of the relaxation
rate was observed around the transition temperatures. This `abrupt'
onset of magnetic order has been observed in other one-dimensional
systems, such as antiferromagnets Sr2CuO3 [55],
Ca2CuO3 [58] and a spin-density-wave system
(TMTSF)2-PF6 [59]. In these systems, the absence
of critical slowing down have been explained as follows; in
one-dimensional systems, the ordering temperature is determined by the
small inter-chain interactions, and therefore, there may be preformed short-range
ordered regions before the three-dimensional order takes
place [60]. Then the ordering process is a
phase-lockings of these short-range ordered regions, which do not
involve a slowing down of the moments. The absence of the
critical slowing down in the 3-leg ladder system may also reflect its
low-dimensionality, if the `phase-locking' picture of the magnetic
order is relevant.
SR spectra at long times. No enhancement of the relaxation
rate was observed around the transition temperatures. This `abrupt'
onset of magnetic order has been observed in other one-dimensional
systems, such as antiferromagnets Sr2CuO3 [55],
Ca2CuO3 [58] and a spin-density-wave system
(TMTSF)2-PF6 [59]. In these systems, the absence
of critical slowing down have been explained as follows; in
one-dimensional systems, the ordering temperature is determined by the
small inter-chain interactions, and therefore, there may be preformed short-range
ordered regions before the three-dimensional order takes
place [60]. Then the ordering process is a
phase-lockings of these short-range ordered regions, which do not
involve a slowing down of the moments. The absence of the
critical slowing down in the 3-leg ladder system may also reflect its
low-dimensionality, if the `phase-locking' picture of the magnetic
order is relevant.
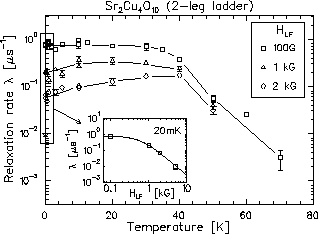
Figure 31:
LF=100 G  SR spectra around the transition temperatures.
SR spectra around the transition temperatures.
 |




Next: 4.3.2 The 2-leg ladder
Up: 4.3 SR measurements
Previous: Longitudinal-field measurements
![]() SR time window (
SR time window (![]() s). In Fig.31,
we show
s). In Fig.31,
we show ![]() SR spectra at long times. No enhancement of the relaxation
rate was observed around the transition temperatures. This `abrupt'
onset of magnetic order has been observed in other one-dimensional
systems, such as antiferromagnets Sr2CuO3 [55],
Ca2CuO3 [58] and a spin-density-wave system
(TMTSF)2-PF6 [59]. In these systems, the absence
of critical slowing down have been explained as follows; in
one-dimensional systems, the ordering temperature is determined by the
small inter-chain interactions, and therefore, there may be preformed short-range
ordered regions before the three-dimensional order takes
place [60]. Then the ordering process is a
phase-lockings of these short-range ordered regions, which do not
involve a slowing down of the moments. The absence of the
critical slowing down in the 3-leg ladder system may also reflect its
low-dimensionality, if the `phase-locking' picture of the magnetic
order is relevant.
SR spectra at long times. No enhancement of the relaxation
rate was observed around the transition temperatures. This `abrupt'
onset of magnetic order has been observed in other one-dimensional
systems, such as antiferromagnets Sr2CuO3 [55],
Ca2CuO3 [58] and a spin-density-wave system
(TMTSF)2-PF6 [59]. In these systems, the absence
of critical slowing down have been explained as follows; in
one-dimensional systems, the ordering temperature is determined by the
small inter-chain interactions, and therefore, there may be preformed short-range
ordered regions before the three-dimensional order takes
place [60]. Then the ordering process is a
phase-lockings of these short-range ordered regions, which do not
involve a slowing down of the moments. The absence of the
critical slowing down in the 3-leg ladder system may also reflect its
low-dimensionality, if the `phase-locking' picture of the magnetic
order is relevant.