In the previous section, the Lorentzian theory was
introduced as a muon spin relaxation theory for dilute spin systems.
This theory is based on the Lorentzian field distribution at the muon
sites. Still, a truly Lorentzian distribution is unphysical, because some
fraction of the muons must locate at an infinitesimally small distance from a
magnetic ion, in order to realize the diverging second moment of the
Lorentzian distribution ![]() . To restore the physicality of the local field distribution,
it will be natural to introduce a large cut-off field
(
. To restore the physicality of the local field distribution,
it will be natural to introduce a large cut-off field
(![]() ) to the Lorentzian distribution. This idea is easily
formulated in the Gaussian decomposed picture of the Lorentzian
distribution (eq.29,30), which has been
introduced to obtain the dynamical Lorentzian relaxation function
) to the Lorentzian distribution. This idea is easily
formulated in the Gaussian decomposed picture of the Lorentzian
distribution (eq.29,30), which has been
introduced to obtain the dynamical Lorentzian relaxation function
![]() .
.
In this picture, a weighting function ![]() was introduced
to sum up the contributions from every muon sites (see
eq.29). In real spin systems, the upper bound of the
site-sum integral should be replaced by a cut-off field
was introduced
to sum up the contributions from every muon sites (see
eq.29). In real spin systems, the upper bound of the
site-sum integral should be replaced by a cut-off field ![]() :
:
 is normalized for the new upper bound
is normalized for the new upper bound
 |
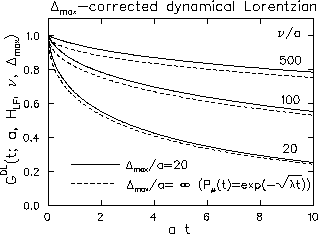
The most significant correction because of the cut-off field
![]() appears in the fast fluctuation regime. In the traditional
Lorentzian theory, the relaxation function in this regime is a
square-root exponential function (eq.32). When the
cut-off field is introduced, the relaxation at large fluctuation rates
loses the fast front-end of the square-root exponential behavior,
because the fast front-end originates from the T1
relaxation of muons which locate at sites with large local fields. In
Fig.22, the
appears in the fast fluctuation regime. In the traditional
Lorentzian theory, the relaxation function in this regime is a
square-root exponential function (eq.32). When the
cut-off field is introduced, the relaxation at large fluctuation rates
loses the fast front-end of the square-root exponential behavior,
because the fast front-end originates from the T1
relaxation of muons which locate at sites with large local fields. In
Fig.22, the ![]() -corrected dynamical
Lorentzian functions [
-corrected dynamical
Lorentzian functions [![]() ]
are compared with the square-root exponential functions of the ideal case.
]
are compared with the square-root exponential functions of the ideal case.
The ![]() -corrected dynamical Lorentzian function
is well approximated by a `stretched' exponential
function,
-corrected dynamical Lorentzian function
is well approximated by a `stretched' exponential
function, ![]() ; in Fig.23, the
stretching power (
; in Fig.23, the
stretching power (![]() ) is shown as a function of the normalized
fluctuation rate (
) is shown as a function of the normalized
fluctuation rate ( ). At small fluctuation rates, the power
converges to 1/2, as expected for the square-root exponential behavior,
and in the large fluctuation limit,
). At small fluctuation rates, the power
converges to 1/2, as expected for the square-root exponential behavior,
and in the large fluctuation limit, ![]() approaches 1.
approaches 1.
 |
Experimentally, muon spin relaxation in paramagnetic dilute spin
systems often exhibits a stretched exponential behavior, with its
power ![]() approaching 1 at high temperatures
[40]. The above mentioned cut-off field effect may
explain at least part of the phenomena.
approaching 1 at high temperatures
[40]. The above mentioned cut-off field effect may
explain at least part of the phenomena.