


Next: A.1 Transverse Field
Up: G.D. Morris' Ph.D. Thesis
Previous: References
In this Appendix we derive from first principles the
eigenvalues and states of muonium, in the simplest case of free
muonium in vacuum with a static magnetic field applied.
The polarization functions with the applied magnetic
field oriented either transverse to or along the initial muon
spin are calculated. Spin relaxation is not considered in this
calculation.
In the situation being considered the Hamiltionian of the muonium atom,
| 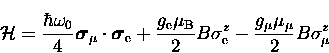 |
(1) |
includes the Zeeman
terms for the muon and electron spins, and the interaction
between these spins.
Table A.2:
Muon and electron g-factors and magnetic moments.
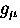 = 2.002331846 = 2.002331846
|
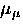 = = 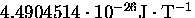
|
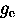 = 2.002319304386 = 2.002319304386
|
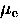 = = 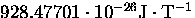
|
In order to determine the time dependence of the muon spin polarization
we diagonalize this Hamiltonian and calculate the elements of
the muon spin operator.
In doing so we will obtain the muonium states and corresponding
energies, which determine the precession frequencies observed in the muon
spin polarization signal.
We start by defining a set of states of the muonium atom
in terms of the individual electron and muon states,
the superscripts on 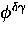 referring to
the muon and electron spins projected
onto the quantization axis, which we take to be the
referring to
the muon and electron spins projected
onto the quantization axis, which we take to be the 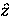 direction.
direction.
The spin operator 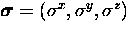 has components given by the usual Pauli matrices
and the muon and electron spin operators act only on their
corresponding parts of the muonium wave function.
has components given by the usual Pauli matrices
and the muon and electron spin operators act only on their
corresponding parts of the muonium wave function.
The operator in the first term of the Hamiltonian
| 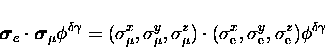 |
(2) |
can be written in terms of raising and lowering operators which are
defined by
by expanding its first two terms into
and similarly
The third term follows trivially from the definition of the spin
along the quantization axis for both the muon and electron,
The hyperfine interaction term is then the sum of
Eqs. (A.5,A.6,A.7)
and we obtain
Finally, noting that
| 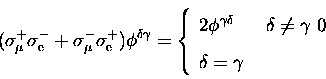 |
(3) |
Eq. (A.8) simplifies to
| 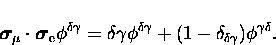 |
(4) |
For the two 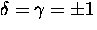 cases it then immediately follows that
the eigenvalues are given by
cases it then immediately follows that
the eigenvalues are given by
and
where we have defined
| 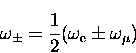 |
(5) |
with
In order to diagonalize the Hamiltonian in the other two cases
where 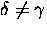 we define linear combinations of the basis vectors
we define linear combinations of the basis vectors
| 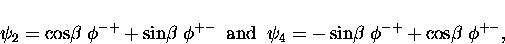 |
(6) |
where the parameter 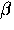 turns out to depend on the magnitude of the field
B, as will be shown below.
turns out to depend on the magnitude of the field
B, as will be shown below.
Operating with the full Hamiltonian on the first of these, we obtain
If 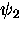 is to be an eigenfunction of the Hamiltonian we must
have both
is to be an eigenfunction of the Hamiltonian we must
have both
where C is an arbitrary constant and we have defined
| 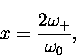 |
(7) |
from which it follows
| ![\begin{displaymath}
x=\left[\tan (2\beta)\right]^{-1}.\end{displaymath}](img325.gif) |
(8) |
Equation (A.18) can now be simplified to
| 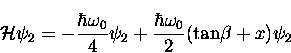 |
(9) |
and by using the trigonmetric identity
| 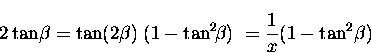 |
(10) |
to substitute
we obtain the final result
| ![\begin{displaymath}
{\cal H} \psi_2 = \left[-\frac{\hbar\omega_0}{4} + \hbar(\om...
...^2/4 +
\omega_+^2)^{1/2} \right]\psi_2 = \hbar \omega_2 \psi_2.\end{displaymath}](img328.gif) |
(11) |
A similar calculation, operating on the wavefunction 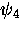 , yields
, yields
| ![\begin{displaymath}
{\cal H} \psi_4 = \left[-\frac{\hbar\omega_0}{4} - \hbar(\om...
.../4 +
\omega_+^2)^{1/2}\right] \psi_4 = \hbar \omega_{4} \psi_4.\end{displaymath}](img330.gif) |
(12) |
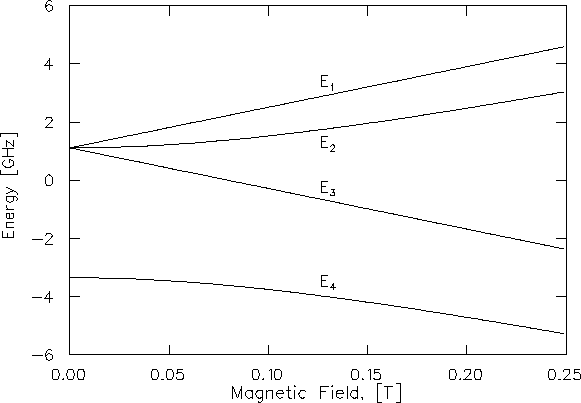
The results so far are summarized in Figure A.32,
which shows the four eigenvalues as functions of the magnetic field B.
Figure A.32:
Breit-Rabi energy level diagram of the four
states of muonium in a magnetic field.
 |
We shall see that the polarization signal has
components at frequencies determined by the transition
energies 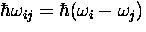 between these states. These frequencies are
between these states. These frequencies are



Next: A.1 Transverse Field
Up: G.D. Morris' Ph.D. Thesis
Previous: References
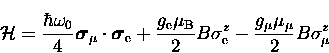
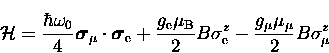
![]() has components given by the usual Pauli matrices
and the muon and electron spin operators act only on their
corresponding parts of the muonium wave function.
has components given by the usual Pauli matrices
and the muon and electron spin operators act only on their
corresponding parts of the muonium wave function.
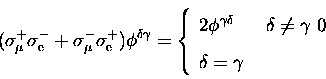
![]() cases it then immediately follows that
the eigenvalues are given by
cases it then immediately follows that
the eigenvalues are given by
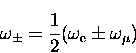
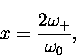
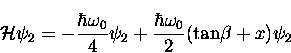
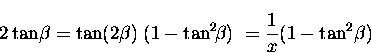
![\begin{displaymath}
{\cal H} \psi_2 = \left[-\frac{\hbar\omega_0}{4} + \hbar(\om...
...^2/4 +
\omega_+^2)^{1/2} \right]\psi_2 = \hbar \omega_2 \psi_2.\end{displaymath}](img328.gif)
![]() , yields
, yields
![\begin{displaymath}
{\cal H} \psi_4 = \left[-\frac{\hbar\omega_0}{4} - \hbar(\om...
.../4 +
\omega_+^2)^{1/2}\right] \psi_4 = \hbar \omega_{4} \psi_4.\end{displaymath}](img330.gif)
![]() between these states. These frequencies are
between these states. These frequencies are