Next: Intrinsic Angular Momentum
Up: Orbital Angular Momentum
Previous: Back to Bohr
The reason 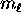 is called the magnetic quantum number
(and the reason m is used for it, rather than some other letter)
is that when one imposes a magnetic field
is called the magnetic quantum number
(and the reason m is used for it, rather than some other letter)
is that when one imposes a magnetic field 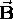 on an atom, the energy levels
on an atom, the energy levels 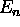 (determined only by n in the
absence of
(determined only by n in the
absence of 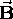 ) are `` split'' by the Zeeman energy
) are `` split'' by the Zeeman energy
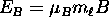 due to the interaction
potential of the magnetic moment with the field (see above).
due to the interaction
potential of the magnetic moment with the field (see above).
In 1925 Goudsmit and Uhlenbeck reported that, in addition to
the ``splittings'' predicted by the quantization of the orbital
angular momentum eigenstates of the electrons in an applied
magnetic field, there were additional splittings
of roughly the same magnitude that could only be explained
in terms of some ``extra'' angular momentum associated with
the electrons themselves. This was relevant to a
previous result that had mystified the community:
In 1922, Stern and Gerlach had done an experiment
on various neutral atoms passing through a region
of large magnetic field gradient, the effect of which
is to exert on the passing atoms a net force
that is proportional to the component of their
angular momentum along the axis of the gradient.
This allowed Stern and Gerlach to experimentally verify
that ``spin 1'' atoms (with 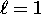 ) did indeed
have three and only three possible values of
) did indeed
have three and only three possible values of
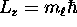 :
: 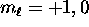 or -1;
and similarly for other integer
or -1;
and similarly for other integer 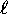 .
However, their experiments on neutral silver atoms
revealed two possible projections of the
angular momentum along the z axis, a range of options
incompatible with the rules
.
However, their experiments on neutral silver atoms
revealed two possible projections of the
angular momentum along the z axis, a range of options
incompatible with the rules 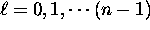 and
and 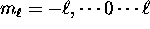 .
The discoveries of Goudsmit and Uhlenbeck suggested that
the electron itself might have an intrinsic
angular momentum that was (somehow) half
as large as the smallest allowable nonzero orbital
angular momentum - what we now call ``spin
.
The discoveries of Goudsmit and Uhlenbeck suggested that
the electron itself might have an intrinsic
angular momentum that was (somehow) half
as large as the smallest allowable nonzero orbital
angular momentum - what we now call ``spin 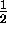 .''
.''
Next: Intrinsic Angular Momentum
Up: Orbital Angular Momentum
Previous: Back to Bohr
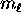 is called the magnetic quantum number
(and the reason m is used for it, rather than some other letter)
is that when one imposes a magnetic field
is called the magnetic quantum number
(and the reason m is used for it, rather than some other letter)
is that when one imposes a magnetic field 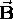 on an atom, the energy levels
on an atom, the energy levels 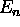 (determined only by n in the
absence of
(determined only by n in the
absence of 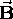 ) are `` split'' by the Zeeman energy
) are `` split'' by the Zeeman energy
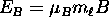 due to the interaction
potential of the magnetic moment with the field (see above).
due to the interaction
potential of the magnetic moment with the field (see above).
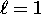 ) did indeed
have three and only three possible values of
) did indeed
have three and only three possible values of
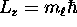 :
: 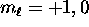 or -1;
and similarly for other integer
or -1;
and similarly for other integer 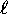 .
However, their experiments on neutral silver atoms
revealed two possible projections of the
angular momentum along the z axis, a range of options
incompatible with the rules
.
However, their experiments on neutral silver atoms
revealed two possible projections of the
angular momentum along the z axis, a range of options
incompatible with the rules 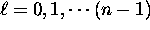 and
and 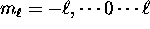 .
The discoveries of Goudsmit and Uhlenbeck suggested that
the electron itself might have an intrinsic
angular momentum that was (somehow) half
as large as the smallest allowable nonzero orbital
angular momentum - what we now call ``spin
.
The discoveries of Goudsmit and Uhlenbeck suggested that
the electron itself might have an intrinsic
angular momentum that was (somehow) half
as large as the smallest allowable nonzero orbital
angular momentum - what we now call ``spin 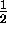 .''
.''